Horizontal launch is an example of composition of motion in two dimensions: an u.r.m. on the horizontal axis and a u.a.r.m. in the vertical one. In this section, we will see:
- The concept and the representation of the horizontal launch
- Its formulas
- The equations of position in the horizontal launch
Are you ready?
Concept and representation
Horizontal launch consists of horizontally launching a body , also know as projectile, from a certain height. In the figure below you can see a representation of the situation:
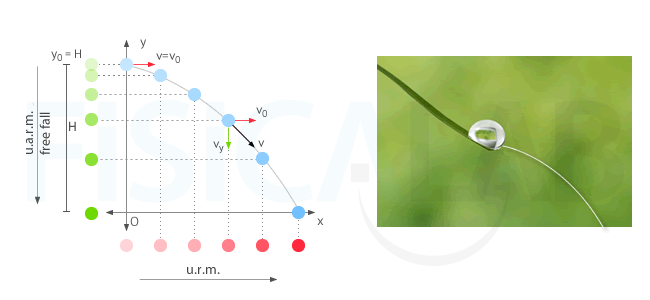
Horizontal launch
Think about a drop that slides at constant velocity (v0) on a leaf located at a height H, when it reaches the edge and falls to the ground. During the fall, it moves at constant velocity v0 in the x-axis (u.r.m.) and it moves in free fall along the y-axis (u.a.r.m.) due to the action of the gravity. Initially, the velocity in this y-axis is 0 (vy = 0) and increases as the projectile descends.
Notice the projections of the motion in the axes and verify that they coincide with the motions that we have described (u.r.m. and u.a.r.m.)
Horizontal launch is the composition of a uniform rectilinear motion (horizontal urm) and a uniformly accelerated rectilinear motion of free fall (vertical uarm). The moving object in this kind of motion is often referred to as projectile, or horizontally launched projectile.
Equations
The equations for horizontal launch are:
-
The equations for the u.r.m. for the x-axis
-
The equations of u.a.r.m. for the y-axis
Since, as stated above, the velocity forms an angle α with the horizontal, the components x and y are determined by using the most common trigonometric relationships:
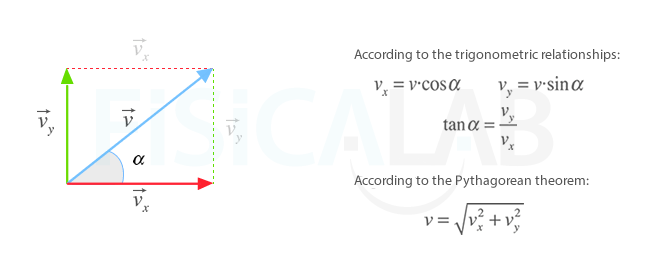
Decomposition of the velocity vector
Any vector, including the velocity, can be broken down is in 2 vectors, vx and vy, that have the same directions as the Cartesian axes. The magnitude of both vectors can be calculated from the angle that the vector forms with the horizontal through the expressions shown in the figure.
Finally, taking into consideration what we previously stated, that y0 = H , x0 = 0 and ay = -g, we can rewrite the formulas as they are shown in the following table. These are the final expressions for the calculation of a horizontally launched projectile kinematic magnitudes:
| Position (m) | Velocity (m/s) | Acceleration (m/s2) | |
|---|---|---|---|
| Horizontal Axis | |||
| Vertical Axis |
Equation of position and trajectory in horizontal launched projectile
The equation of position of a body helps us to determine at what point it is in each moment in time. In the case of a body that is moving in two dimensions, remember that, generically, displacement is described by:
Substituting in the previous expressions of the position in the horizontal axis (u.r.m.) and on the vertical axis (u.a.r.m.) in the generic equation of position, we can get to the expression of the equation of position for a horizontal launched projectile.
The equation of position for a horizontal launched projectile is given by:
On the other hand, to determine the trajectory that the body follows, i.e., its trajectory equation, we can combine the previous equations to eliminate t, leaving:
Where

