Solved exercises worksheet
Test what you have learned in the subject Motion in Physics with this list of exercises with their respective solutions and classified by sections.
Motions and Reference Frames
Reference Frames in Kinematics
Say whether the following statements are true or false:
a) Reference frames can be classified as inertial and heterogeneous.
b) The study of motion is independent of the observer´s position in relation to the motion.
Position Vector
What is the position vector?
Find the position vector and its magnitude for the following points:
a) P1 (-1,2)
b) P2 (-3,4)
Trajectory and Equation of Position
Position Equation of the S.H.S. Madrid-Barcelona (Spain High Speed train)
The S.H.S. Madrid-Barcelona during the first stage of its trip travels east at a rate of 50 m each second and North at 30 m each second. Calculate:
- The motion x-coordinate as function of time
- The motion y-coordinate as a function of time
- The position equation of the train for the studied stretch
- The distance to the origin of the section as a function of time
Distance Traveled
Crescent Shaped Motion
A body moves between any two instants of time following a circular path with a radius of 5 meter, as you can see in the figure.

Determine:
- The displacement vector of the body and the distance traveled, assuming the origin of the system of reference is located at the starting point of the motion
- The displacement vector of the body and the distance traveled, assuming the origin of the system of reference is in the center of the semicircle
Difference between Displacement and Distance Traveled
What do you know about displacement and distance traveled?
Answer if the following statements are true or false:
a) Distance traveled only depends on the starting and final positions without consideration of the trajectory.
b) Distance traveled is always greater or equal than the magnitude of the displacement vector.
c) Distance traveled it is a vector.
d) As time passes, a body in motion always increases its displacement.
Average Velocity
Calculation of average velocity
If a body is in the position (1,2) and after 2 seconds it is in the position (1,-2).
What will be its average velocity considering that all units are expressed in the International System?
Instantaneous Velocity
Velocity starting with the position equation
If a body moves according to the following equation:
Calculate its instantaneous velocity at time t=1 s.
A particle in motion
The position of a certain particle depends on time according to the equation
- Determine the displacement and the average velocity during the interval 3.0s ≤ t ≤ 4.0 s.
- Find the general formula for the displacement for the interval between t and t + Δt.
- Find the instantaneous velocity for any time t considering the limit whenΔt approaches 0.
Average Speed
Average speed of the Moon
Knowing that the Moon takes 28 days to make one complete revolution around the Earth, calculate its average speed.
Datum: Consider the trajectory of the Moon as circular with a radius of 384,000 Km.
Average speed in a circular trajectory
A body is in a circular track like the one in the figure.

If the radius is 80 m, calculate:
- The average speed between points A and B, if the body takes 13 seconds to go from one point to the other
- The average speed between points A and C if a cyclist takes 26 seconds to go from one point to the other
- The average speed between point A and C if the rider takes 13 seconds to go from point A to point B
Average speed in a full circle
A body does a full circle of 4-meter radius in 1.5 seconds. Calculate:
a) the average speed.
b) the average velocity.
Instantaneous Speed
Speed as a function of distance traveled
Knowing that the distance a body travels as a function of time is given by the following equation:
Calculate:
a) The average speed during the first 3 seconds.
b) The instantaneous speed of the body.
Concept of Acceleration
Acceleration graphs
Given the following graphs that show the variation of the speed with time of three different bodies, answer the following questions:
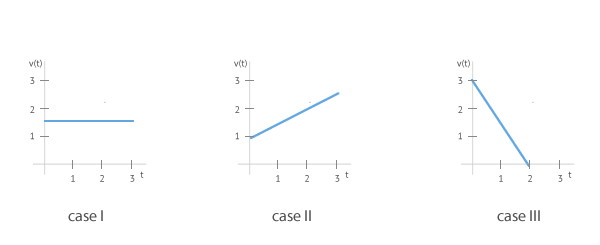
a) Which case has the fastest variation of the speed?
b) What does the slope represent in each one of the 3 cases?
c) Why is the line in case I horizontal?
d) In case I, does the possibility exist that the body is experiencing acceleration?
Average Acceleration
Acceleration in a basketball throw
A basketball player throws the ball with a velocity of
Calculate the average acceleration knowing that the impact against the board lasts exactly 0.02 seconds
Instantaneous Acceleration
Acceleration of a meteorite
A meteorite moves through space with a velocity v⃗(t) = (1+4·t) i⃗+t2 j⃗ m. Calculate
a) Its average acceleration between the times t1=2 s and t2= 4 s.
b) Its acceleration at t3= 6 s.
Tangential Acceleration
Magnitude of the tangential acceleration
Knowing that the magnitude of the velocity of a body in S.I. units is:
Calculate the magnitude of the tangential acceleration.
Types of Motions According to the Acceleration
Identifying motion
Answer if the following statements are true or false:
a) If motion has normal acceleration, the motion is rectilinear.
b) If motion has tangential acceleration, the motion is curvilinear.
c) Normal and tangential acceleration are called intrinsic components of the acceleration.
d) Motion without normal acceleration and with constant tangential acceleration is called uniformly accelerated rectilinear motion. (u.a.r.m.)
Rectilinear Motion: Sign Convention
Conversion of vectors to scalars in rectilinear motion
Assuming that the following vector magnitudes refer to rectilinear motion, give their corresponding scalar representation:
Equations of Constant Velocity Motion
u.r.m. in collision marbles
Two marble players face each other with their marbles in hand. The game consists of throwing the marbles at the same time in a straight line so they hit each other. The players are located 36 meters from each other and player A launches its marble at 2 m/s and player B at 4 m/s, in a uniform rectilinear motion. Calculate that distance from player B at which the marbles will collide.
Time at which two bodies with u.r.m. will meet
Two bodies depart from the same point in the same direction, with uniform rectilinear motion. Knowing that they depart 15 seconds apart, that the first one does it at a speed of 20 m/s and the second one at a speed of 24 m/s, determine at which time they will meet and how far from the origin.
u.r.m. velocity in the collision of two bodies
Two bodies depart with 15 s of difference from the same point in the same direction. Knowing that the speed of the first one is 72 km/h, what should be the speed of the second to reach it in 90 s?
Note: You can assume that both bodies move with uniform rectilinear motion.
Constant Velocity Motion Graphs
Draw graphs from u.r.m. equations
Determine the graphs of the following uniform rectilinear motions:
- x = 3 + 4·t
- x = 3 - 4·t
- x = -3 + 4·t
- x = -3 - 4·t
- 3·x = 9 + 12·t
Where x is measured in meters and t in seconds.
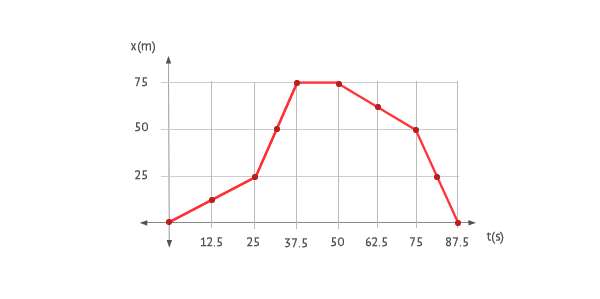
x-t graph of a person walking
Analysis of uniform rectilinear motion graphs
The following graph of position-time ( x-t ) corresponds to a body moving in a straight trajectory. Determine the equation of motion for each segment and its velocity-time graph. From the expression for each segment, search for a general expression in the form of a function defined by segments, called a piecewise-defined function, for the position and one for the velocity.
Equations of Constant Acceleration Motion
A bicycle with constant acceleration
A cyclist starts his morning ride and after 10 seconds his velocity is 7.2 km/h. At that moment, he sees a dog approaching and slows down for 6 seconds until the bicycle stops. Calculate:
a) The acceleration until he begins to slow down.
b) The braking acceleration of the bike.
c) The total distance traveled.
Free Fall
Gravity on the Moon
Determine the acceleration of gravity on the Moon knowing that if you drop an object from a height of 5 m, it takes 2.47 s to reach the ground.
Glass in free fall
A glass of water on the edge of a table falls towards the floor from a height of 1.5 m. Considering that gravity is 10 m/s2, calculate:
a) The time the glass is in the air.
b) The velocity with which it impacts on the ground.
Free fall in a well
Determine the depth of a well in which a stone is dropped, and where you can hear the impact on the water after 1.5 s, considering that the velocity of sound is 340 m/s.
Drops in fall free
A broken faucet lets out water drops every 1/4 of a second. If the faucet is just 3 meters above the ground, and if one drop falls in this very instant, determine what is the position of the drops still in the air at this moment.
Free fall from unknown height
Vertical Launch
A rookie equilibrist
A rookie equilibrist is standing on a platform 12 meters above the ground. While practicing juggling with 2 balls, he stumbles and throws both balls at 9 m/s, however, he throws one up which we will call A and the other one down which we will call B. Considering that gravity is 10 m/s2, calculate
a) The time they are in the air.
b) Their velocity when they hit the ground.
c) The maximum height that ball A reaches.
A launch of negligible mass
From a height of 40 meters an object of negligible mass is thrown downward with a velocity of 20 m/s. How long will it take to hit the ground? What will be its velocity on impact?
Vertical launch and free fall
A stone is let to free fall to the bottom of a cliff with a height of 80 m. A second later a second stone is thrown downward so that it reaches the bottom at the same time as the first one
- What was the launch velocity of the second stone?
- What was the velocity of the first stone when they both hit the bottom?
- How long was the second stone in the air?

